Gradient evaluation on non-orthogonal meshes
Jose Caravaca Fernandez, Leibniz Universität Hannover,
Seminar
07/2011
The gradient of a scalar function is a well-known tool of vector analysis and routinely used to analyze images or results of simulations or to enhance volume visualization.
In these applications, the gradient is usually not known but has to be approximated from the values of the function which are commonly given on a discrete set of points in some space. If these points lie on a regular, orthogonal grid, approximation of the gradient can be easily done using divided differences.
In the case of non-orthogonal meshes, this is not as simple. In this seminar, several methods will be presented which can overcome this problem.
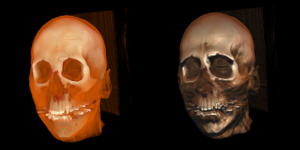
Volume raycasting without (left) and with (right) gradient hinting.
Contact: Philipp Blanke